In the first post in this series, I talked about the difference between solving problems and problem solving. This week, I will continue my series on problem solving and share five essential problem solving techniques for your problem solving routines.

A strong problem-solving routine is essential for helping students develop their problem-solving strategy toolboxes. Over the years, I have used a variety of routines that have helped my students develop problem-solving strategies and critical thinking skills. (Read more about my favorite routine here!) Through a lot of trial and error, I found several routines that worked well for my students. (I will share more about them next week!) Today, I want to share some trade secrets with you to help you get the most from your problem-solving routines with five essential problem-solving techniques.
Five Essential Problem Solving Techniques
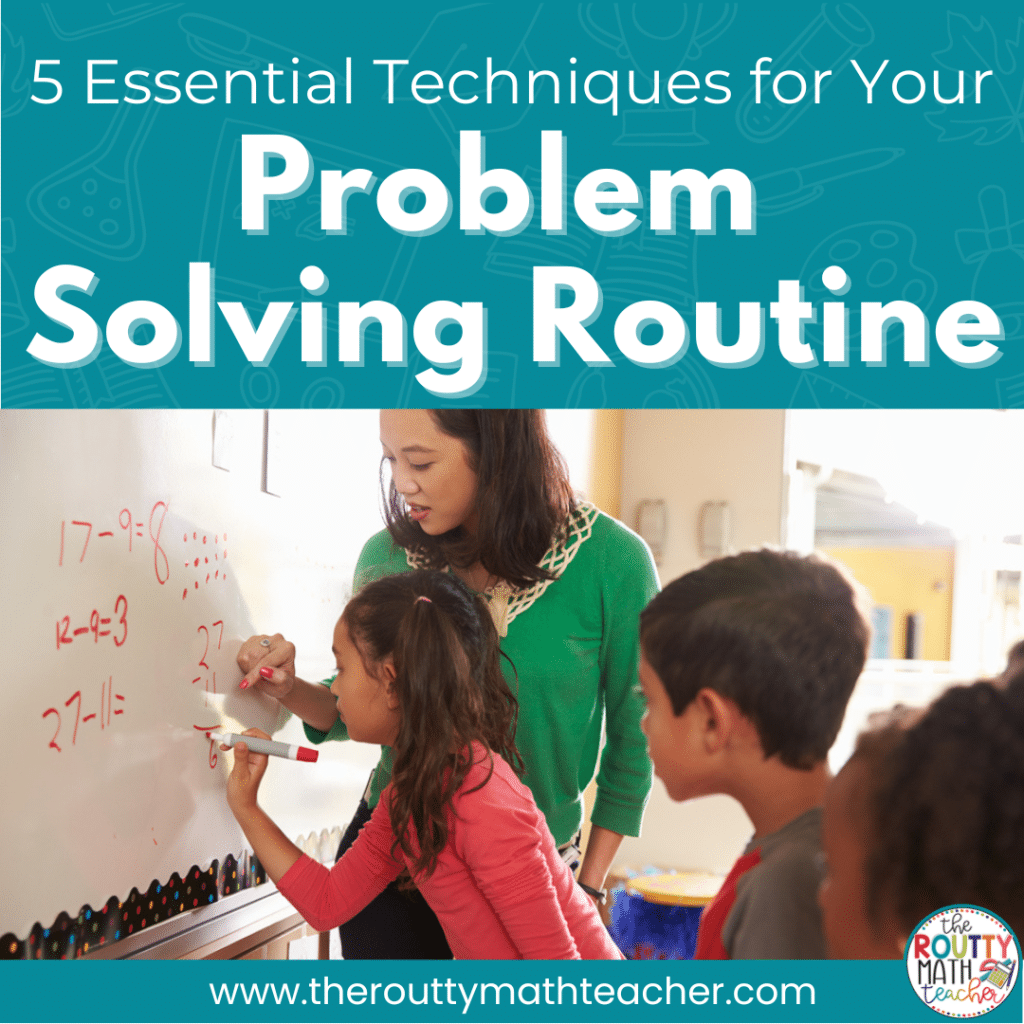
1. Share student thinking and strategies.
This is essential! I can’t tell you how many times I have seen teachers give a great problem solving or critical thinking task and then never allow students to share their responses. Sometimes, our students are the best teachers and they can get a message across when we struggle to do so. Also, providing an opportunity for students to talk to other students about their thinking increases math vocabulary and builds communication skills.
After students have had an opportunity to share their thinking with a group member or partner, I encourage you to discuss the task as a class. This gives the teacher an opportunity to reiterate correct thinking, modify incorrect thinking, ask questions, build math vocabulary, and increase students’ communication skills.
Read more about getting started with math talk in the classroom here.
2. Solve non-routine problems.
In an earlier blog post, I emphasized the importance of using non-routine problems with students. Not only are students typically more engaged, but students have the opportunity to use strategies beyond writing an equation/number sentence or drawing a picture. If you’re interested in some fun, non-routine tasks, please check out my Solve It! Friday page.
One of the things many people say they love about math is the fact that there is a right and wrong answer. While there certainly are wrong answers, sometimes, there can be more than one right answer. These types of tasks really stretch some kids’ thinking. They also provide a natural venue for discussion. Students can debate the answers only to discover that more than one works!
3. Discuss efficiency.
During problem-solving experiences, students will often use beautiful and complicated solution strategies to solve problems. While we want to encourage outside-of-the-box thinking, we also want students to attend to efficiency. One way to do this is to have several students share their solutions. They can then discuss what strategies are best for specific types of problems. When discussing difficulty becomes a regular part of your routine, students will begin to utilize their problem-solving strategies in a way that not only gets them to the correct answer but also using an efficient method.
4. Make connections.
Recently, I wrote about making connections as part of my Summer PD series. Read it here! When students make connects, it deepens their understanding of other content and skills. One way to do this is to connect the problem-solving task to grade-level content and skills. Another way is to have students represent problems in a variety of ways, i.e. pictures, numbers, words, or equations. Each representation is crafted in a specific way, so being able to translate words into an equation or numbers into a picture is a big skill that has many benefits.
5. Use “high ceiling, low floor tasks.”
The term “high ceiling, low floor” refers to a task having multiple entry points to allow all students a way to access the task; however, it also includes ways to extend the tasks for those students who are ready for more of a challenge. These types of tasks increase participation because students can participate at a level that is comfortable for them. Students are also able to showcase what they can do instead of what they are unable to do. Even better, these tasks provide instant opportunities for differentiation because all students can participate in a way that allows them to be most successful.
Using a regular problem-solving routine can help students develop the tools necessary to be powerful thinkers of mathematics; however, in order to get the most from the routines, certain problem-solving techniques must be included. While you may not want to add all of the above techniques to your routine, I encourage you to commit to adding one or two of them this year. I highly recommended starting with “sharing student thinking and strategies.” It’s probably the most important technique of all of the problem-solving routines. It will get you the most bang for your buck!
Sound Off! How do get the most from your problem-solving routine? Which problem-solving techniques do you think are most important?
Respond in the comments below. 






3 Responses
Hi, can you provide an example of a high ceiling, low floor task? Thank you!
Hi Jen!
Great question! The high ceiling, low floor tasks give all students a chance to engage in the task but have places to go to extend the learning for students. One problem that comes to mind is a task where students are asked to find combinations of numbers to achieve a goal, like the following problem: Farmer Brown’s niece Angie is in charge of her uncle’s farm while he is on vacation. He gave her strict instructions to make sure none of the animals ran away. When Angie counted the pigs and chickens, she counted 32 legs. How many pigs and chickens did she count?
All students should be able to determine a combination of pigs and chickens; however, what if I added a new condition to say: Angie counted a total of 12 animals.
This changes the level of rigor because students are now looking for a specific combination. Some students will struggle with this but others may be ready to tackle it; so, using tasks that have a high-ceiling allow for this flexibility.
Check out more high ceiling, low floor tasks here: https://www.youcubed.org/task-grades/low-floor-high-ceiling/.
Shametria
Thank you!