As teachers, we know one of the most challenging aspects of teaching is meeting the needs of a variety of learners. In today’s post, I’m sharing seven ways to differentiate learning for your gifted and talented students.
My most challenging year in the classroom was when I taught the gifted and talented class.
I know many people had to re-read that statement, but it’s true.
Getting to the nitty-gritty of math content is what I love about teaching math the most. I thrive on discovery and playing with math!
But, as I found out, that’s not how many of my gifted students’ brains worked.
I knew I needed to challenge them, but I wasn’t sure how.
The challenges I used with my students were too easy for my gifted students. What took my non-gifted students several minutes to do could be completed by my gifted students in no time.
I was tempted to teach them higher-level math, but they still needed to learn the expected grade level content too.
It was such a dilemma.
It took some time, years, in fact, to realize I didn’t need to teach more content because my gifted students’ pace of learning was faster, I needed to increase the level of rigor for my gifted and talented students.
Supporting Our Gifted and Talented Students
Each year, 20 to 30 students with a diverse set of needs walk through our doors, from learning styles to varying levels of content knowledge and preparedness to learning accommodations– some of our students come with their own user guide!
Many teachers translate differentiating for these students to mean more work; however, best practice suggests more is not better. In fact, many gifted and talented students dislike the label because it often results in more homework and higher expectations.
While I understand the logic, it’s important we consider common characteristics of gifted and talented learners. Most learners in this group catch on fast, so fewer repetitions and practice are preferred– which directly contradicts the notion that gifted and talented students should receive more work rather than more rigorous work.
But, if more work is not the answer, what is?
Differentiating for Gifted and Talented Students
Just like we do for our lower achieving students or students with disabilities, we differentiate for our gifted students. If you’re like me, you know what to do with the lower achievers and students with disabilities, but you’ve had little experience differentiating for high achievers.
The author of Good Questions: Great Ways to Differentiate Mathematics Instruction in the Standards-Based Classroom offers three elements to effectively differentiate instruction.
- Focus on the big ideas of the unit or lesson.
- Evaluate student understanding, either formally or informally, to determine student needs.
- Provide choice by differentiating the content, process, or product.
Marion Small (2017) states, “to differentiate instruction effectively, teachers need manageable strategies that meet the needs of most of their students at the same time” (p. 6). She recommends the use of two strategies to do this, open questions and parallel tasks.
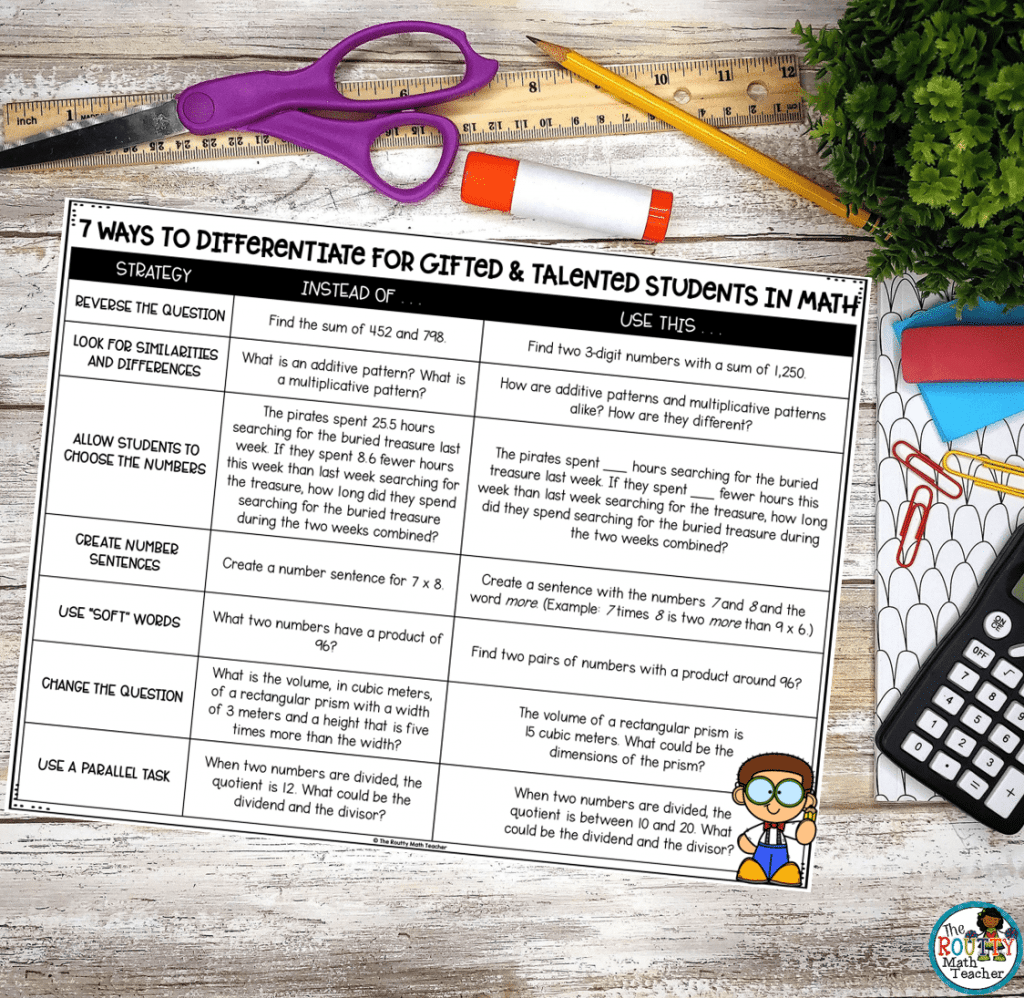
Open Questions
Open questions are those where “a variety of responses or approaches are possible” (Small, p. 7) which allows them to be accessible to a variety of learners.
Strategies for creating open questions include:
- Reverse a question by providing the answer instead of the question. For example, instead of asking for the sum of 452 and 798, give the sum of 1,250 and ask students to provide two, or three, 3-digit addends to equal the sum.
- Analyze similarities and differences. For example, how are additive patterns and multiplicative patterns alike? How are they different?
- Allow students to choose the numbers. For example, provide a word problem without numbers. Then allow students to select the numbers and solve the problem.
- Create number sentences. This could be as simple as rolling a few dice and asking students to create a target number with the numbers rolled or by giving students numbers and words to create a sentence. For example, when given the numbers 7 and 8 and the word more, students may write “the square of 8 is more than the square of 7” or “7 times 8 is two more than 9 x 6″.
- Use “soft” words. This strategy encourages critical thinking about numbers and requires the use of “soft” words instead of absolutes. For example, instead of asking for two numbers with a product of 96, ask for two numbers that have a product around 96.
- Change the question. For this strategy, change up a question you are already using. For example:
- Question 1: What is the volume, in cubic meters, of a rectangular prism with a width of 3 meters and a height that is five times more than the width?
- Question 2: The volume of a rectangular prism is 15 cubic meters. What could be the dimensions of the prism?
Parallel Tasks
Parallel tasks are sets of two or three questions, or tasks, designed to meet the needs of a variety of learners. They are focused on the same big idea and concept but accessible to all students and can be discussed at the same time.
For example:
- Task 1: When two numbers are divided, the quotient is 12. What could be the dividend and the divisor?
- Task 2: When two numbers are divided, the quotient is between 10 and 20. What could be the dividend and the divisor?
When creating parallel tasks, it’s important to consider student abilities, size of numbers, the meaning of operations, etc. In addition, it’s important to consider the questions to be asked of students. The best parallel tasks allow the same set of questions to be used for both tasks so all students can engage in the discussion.
What’s Next?
Through the use of open questions and parallel tasks, differentiating for our gifted and talented students doesn’t have to be daunting. In fact, you can sometimes use the same materials for your gifted learners as you do with the rest of your class with a few modifications. As you prepare your next math unit or lesson, give some of the ideas above a try for your gifted and talented students or high achievers.
Grab your free copy of the differentiation chart using the form below.
Sound Off!
How do you differentiate for your gifted and talented students? Share your thoughts in the comments section below.
References
Small, M. (2017). Good questions: Great ways to differentiate mathematics instruction. New York: Teachers College





27 Responses
Thank you for the resources.
Hello!
It’s my pleasure! Enjoy.
~ Shametria
Thank you for the resources they for sure will come in handy.
Hello!
It’s my plesure. Enjoy!
~ Shametria
Thank you for all the resources!
It’s my pleasure!
~ Shametria
I enjoyed the article, especially the section on differentiating for gifted and talented students.
I’m so glad you found the post helpful, Gabriela!
~ Shametria
These resources are great!!! Thank you!
Thank you for the resources, would like to get more helpful ideas for my GT students.
Great resources!
Thank you for the resources
Thanks for the resources
Thanks for the resources.
Thank you! They will really help my guide instructions to another level.
Thanks for all of the great information!
Thanks for the resources. Always open to new ideas!
These resources are great for GT and the whole class.
Thank you for the resources I will be using them for all my students
Thank you for the resources!
Thanks for the resources!
Thank you for the resources.
Thank you for sharing your expertise with us, I know it would be of great help to us Math teachers.
Thank you for the resources.
Your resources were very informative. Thank you.
Giving advanced students more opportunities to increase their knowledge will encourage them to continue to enjoy learning. Thank you for the information.
Great ideas! I will surely use some of these resources.